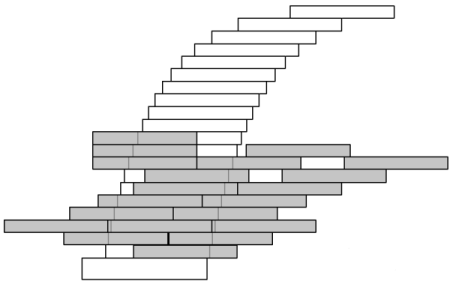
I attended an interesting seminar on the block stacking problem today given by Professor Mike Paterson of the University of Warwick.
Like all good problems, it can be stated simply: how far can a stack of *n* identical blocks be made to hang over the edge of a table?
The answer was widely believed to be of order log n (like the white shaded example above), but Mike Paterson and Uri Zwick previously constructed overhangs of order n^{1/3}.
In the seminar today, Paterson and his co-authors presented their proof that order *n^{1/3}* is indeed the upper bound possible, resolving a long-standing mathematical problem. Professor Paterson is an excellent orator, highly recommended.
